
In addition to conventions, there are some important assumptions about numbers and figures that are listed in the Quantitative Reasoning section directions: Whenever nonstandard notation is used in a question, it is explicitly introduced in the question.
For example, the positive direction of a number line is to the right, distances are nonnegative and prime numbers are greater than 1. The mathematical symbols, terminology and conventions used in the Quantitative Reasoning measure are standard at the high school level. The Math Review (PDF) provides detailed information about the content of the Quantitative Reasoning measure. It doesn’t include trigonometry, calculus or other higher-level mathematics. The content in these areas includes high school mathematics and statistics at a level that is generally no higher than a second course in algebra. These topics are typically taught in high school algebra courses or introductory statistics courses. counting methods, such as combinations, permutations and Venn diagrams.random variables and probability distributions, including normal distributions.elementary probability, such as probabilities of compound events and independent events.interpretation of data in tables and graphs, such as line graphs, bar graphs, circle graphs, boxplots, scatterplots and frequency distributions.basic descriptive statistics, such as mean, median, mode, range, standard deviation, interquartile range, quartiles and percentiles.The ability to construct proofs is not tested. triangles, including isosceles, equilateral and 30°-60°-90° triangles.coordinate geometry, including graphs of functions, equations and inequalities, intercepts and slopes of lines.
setting up equations to solve word problems.

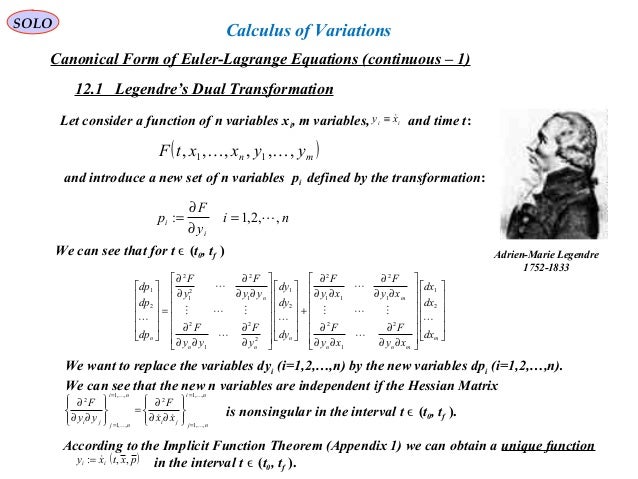
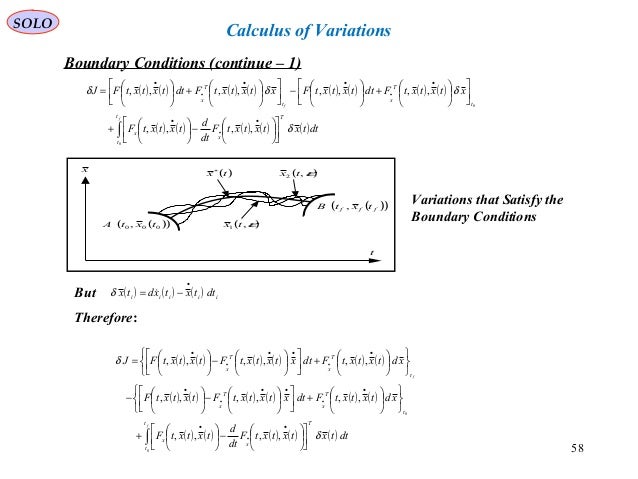
properties and types of integers, such as divisibility, factorization, prime numbers, remainders and odd and even integers.The skills, concepts and abilities are assessed in the four content areas below. Many of the questions are "word problems," which must be translated and modeled mathematically. The Lagrangian and Hamiltonian variational approaches to mechanics are the only approaches that can handle the Theory of Relativity, statistical mechanics, and the dichotomy of philosophical approaches to quantum physics.Some of the Quantitative Reasoning questions are posed in real-life settings, while others are posed in purely mathematical settings. In fact, not only is it an exceedingly powerful alternative approach to the intuitive Newtonian approach in classical mechanics, but Hamilton’s variational principle now is recognized to be more fundamental than Newton’s Laws of Motion. This variational approach is both elegant and beautiful, and has withstood the rigors of experimental confirmation. The calculus of variations provides the mathematics required to determine the path that minimizes the action integral. \) follows a path that minimizes the scalar action integral \(S\) defined as the time integral of the Lagrangian.


 0 kommentar(er)
0 kommentar(er)
